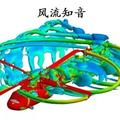
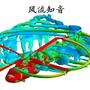
【物理建模】DBM study on Kelvin-Helmholtz instability
风流知音【物理建模】DBM study on Kelvin-Helmholtz instability CFDST(2019)1025
Cover paper in Frontiers of Physics:
Nonequilibrium and morphological characterizations of Kelvin-Helmholtz instability in compressible flows

As an important mechanism of turbulent mixing, the Kelvin-Helmholtz (KH) interface instability, often referred to as KH instability, caused by tangential velocity difference on both sides of the interface, widely exists in the fields of high-energy density physics, earth and astrophysics, inertial confinement fusion, combustion, Bose-Einstein condensation, graphene and so on. For example, in the field of astrophysics, on the one hand, the fully developed KH instability leads to the formation of large-scale vortex structures in the interaction between interstellar hurricanes, galactic spiral arms, solar wind and the earth's magnetosphere. On the other hand, the significantly inhibited KH instability contributes to the formation of high collimation, high length-width ratio and high stability supersonic celestial jets. In the implosion process of inertial confinement fusion, KH instability, as a secondary instability, intensifies the nonlinear development of Rayleigh-Taylor and Richtmyer-Meshkov interface instabilities in the late stage, and improves the speed and degree of mixing of fluids near the interface. In the late stage of deflagration to detonation, the KH instability caused by flame and explosion greatly stimulates turbulent combustion, enhances the mixing efficiency of fuel and flame in the reaction process, and finally promotes the formation of detonation.
Because of its important role in basic science and engineering application, the research on KH instability is increasing. Even so, there are still at least two aspects that need to be strengthened: (i) kinetic modeling, simulation and Thermodynamic Non-Equilibrium (TNE) effect of KH instability, (ii) after obtaining the numerical simulation results, it is still an open subject how to effectively understand and grasp the complex multi-scale spatio-temporal structures and cross-scale associations emerging in the unstable development of KH, and how to effectively extract reliable information from these structures or patterns so as to conduct hierarchical and quantitative research on them. At present, most studies on KH instability are based on macroscopic models that do not fully consider TNE effect, such as Euler or Navier-Stokes equations. However, the different stages of fluid instability development often involve dynamic behaviors and kinetic modes of various scales, which are typically non-linear, non-equilibrium, multi-scale and complex. Especially in the late stage of instability evolution, small scale structures emerge one after another, interscale interfaces are rich and complex, and macroscopic hydrodynamic and thermodynamic non-equilibrium effects are very significant. In addition, accurate simulation of small-scale structures in the system challenges traditional fluid models based on continuum assumptions. Because the mesh precision and time step are limited by the model precision, the mesh size and time step cannot be reduced to the scale that the physical model is no longer valid. When the mean distance between molecules is no longer negligible relative to the scale of the structure under consideration, the continuum hypothesis is no longer reasonable. The traditional fluid model based on continuum hypothesis is not good enough in physical description when it is used to simulate small-scale structure more accurately and fully understand macroscopic flow and thermal non-equilibrium behavior. As we all know, the molecular dynamics method based on the Newton's second law of particle movement, does not rely on continuous medium hypothesis, from molecules (or atoms) level to reveal the non-equilibrium behavior characteristics, mechanism and laws, but the problem is: computational complexity, dependence on the computer's memory of molecular dynamics are extremely high, the actual simulation time and space scale in most cases far cannot satisfy the demand.

Figure 1 DBM simulation of the viscosity effect of KH instability, where (a)-(d) are for the same time, and the corresponding viscosity increases successively.

Figure 2 Viscous effect of KH instability, where (a) and (b) show inhibition of KH. development rate, (c) shows extension of linear stage duration, (d) shows reduction of maximum disturbance kinetic energy.

Figure 3 Viscous effect of KH instability, where (a) and (b) show that it enhances the overall and local TNE intensity, expands the non-equilibrium range, (c) shows that it inhibits the macroscopic flow rate, (b) and (d) show that the TNE strength and interface length exhibit a high spatio-temporal correlation.
The evolution process of KH instability is studied, specifically focusing on the TNE behaviors and effects ignored by traditional fluid modeling and not directly studied by molecular dynamics simulation due to the limitation of applicable space-time scale. At the same time, in order to solve the analysis problems of various complex physical fields in the process of the KH instability evolution, they proposed a new way to conduct physical identification of characteristic structure or pattern and design of tracking scheme through the combination of tracking non-equilibrium behavior characteristics and morphological analysis technology [4-5], and quantitatively characterize the width and development rate of the mixed layer of KH. It is found that the width of the mixed layer, the non-equilibrium strength and the length of the interface boundary are spatio-temporal correlation. Typical non-equilibrium characteristic viscosity, highly correlated with unorganized momentum flow, inhibits the development rate of KH, prolongs the duration of the linear stage, reduces the maximum disturbance kinetic energy, improves the overall and local thermal non-equilibrium intensity, and expands the non-equilibrium range. In general, heat conduction (another characteristic of non-equilibrium that is highly correlated with disorganized energy flow), consistent with the viscous effect, inhibits the small wavelength growth during the instability evolution of KH, making it easier for the system to form large-scale structures. According to the increase of disturbance amplitude, different from the consistent inhibition of viscosity on KH, the stage effect of heat conduction was observed in the case of large heat conduction. Inhibition is due to heat conduction expanding the interface width, reducing the macroscopic gradient and the strength of non-equilibrium driving force, and enhancement is due to the expanded density transition layer, which makes KH easier to absorb energy from the fluids on both sides [6], thus enhancing the instability of KH. The competition of these two effects exists in the whole process of the instability evolution of KH. The inhibition effect dominates in the early stage and the enhancement effect dominates in the late stage.

Figure 4 Heat conduction effect of KH instability: first restraining and then raising.
In addition to the above specific conclusions, the information conveyed in this paper also includes that the DBM based on Boltzmann equation is equivalent to a macroscopic fluid model plus a coarse-grained model for TNE effects. The coarse-grained model can be used to make up for the deficiency of macroscopic fluid model in the description of specific non-equilibrium state or effect. DBM, in terms of the fine degree of physical description, is coarser than the original Boltzmann equation and finer than the Navier-Stokes equations, which are between the two. In terms of the applicable space-time scale, it surpasses microscopic molecular dynamics simulation. It provides a convenient and effective way to study the main non-equilibrium behavior of complex fluid system.
References:
1. Xu A, Zhang G, Zhang Y. Discrete Boltzmann modeling of compressible flows. in: G. Z. Kyzas, A. C. Mitropoulos (Eds.), Kinetic Theory, InTech, Rijeka, 2018, Ch. 02. doi:10.5772/intechopen.70748.
https://www.intechopen.com/books/kinetic-theory/discrete-boltzmann-modeling-of-compressible-flows
2. Gan Y, Xu A, Zhang G, Zhang Y, Succi S. Discrete Boltzmann trans-scale modeling of high-speed compressible flows. Physical Review E 97,053312 (2018).
https://journals.aps.org/pre/abstract/10.1103/PhysRevE.97.053312
3. Xu A, Zhang G, Gan Y, Chen F and Yu X. Lattice Boltzmann modeling and simulation of compressible flows. Frontiers of Physics 7, 582 (2012)
http://journal.hep.com.cn/fop/EN/10.1007/s11467-012-0269-5
4. Xu A, Zhang G, Pan X, Zhang P, and Zhu J. Morphological characterization of shocked porous material. Journal of Physics D: Applied Physics 42, 075409 (2009)
http://dx.doi.org/10.1088/0022-3727/42/7/075409
5. Gan Y, Xu A, Zhang G, Li Y and Li H. Phase separation in thermal systems: A lattice Boltzmann study and morphological characterization. Physical Review E 84, 046715 (2011)
http://dx.doi.org/10.1103/PhysRevE.84.046715
6. Gan Y, Xu A, Zhang G and Li Y. Lattice Boltzmann study on Kelvin-Helmholtz instability: Roles of velocity and density gradients. Physical Review E 83, 056704 (2011)
http://dx.doi.org/10.1103/PhysRevE.83.056704
A similar Chinese version of this brief introduction is referred to FOP 物理学前沿FOP刊 2019年8月23日,